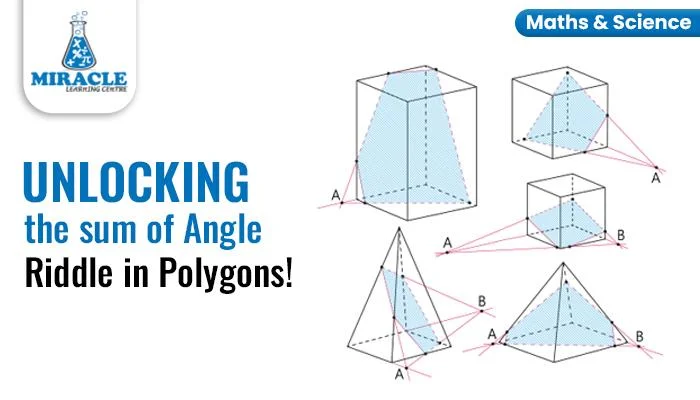
Polygons are fundamental geometric shapes that we encounter in various aspects of our daily lives. From rectangles and triangles to more complex polygons like hexagons and octagons, these shapes are all around us. One intriguing aspect of polygons is the relationship between the number of sides and the sum of their interior angles. Our Maths tuition Centre is dedicated to illuminating the intricate world of mathematics for students of all ages. Today, we embark on a journey to explore the sum of angles in a polygon—a fundamental concept that underpins various mathematical and geometric principles.
Let's delve into this topic, uncover essential formulas, and gain a deeper understanding of the fascinating relationships that govern polygons and their interior angles.
The Basics of Polygons in Maths
Before delving into the sum of angles, let's establish a clear understanding of what polygons are. A polygon is a closed two-dimensional shape with straight sides. The sides do not intersect, and each side connects to the adjacent sides at vertices. The simplest polygon is the triangle, which has three sides and three angles. As we move to polygons with more sides, the complexity increases, but so does our fascination with their properties.
Types of Polygons in Maths:
Polygons exhibit a diverse array of properties, which allows us to classify them into various categories based on their characteristics. These classifications can be based on both the number of sides and other specific properties. Let's explore these categorizations in detail:
Based on the Number of Sides:
- Triangle (3 sides)
- Quadrilateral (4 sides)
- Pentagon (5 sides)
- Hexagon (6 sides)
- Heptagon (7 sides)
- Octagon (8 sides)
- Nonagon (9 sides)
- Decagon (10 sides)
Based on Other Properties, polygons are divided into:
- Regular Polygons and Irregular Polygons
- Concave Polygons and Convex Polygons
- Equilateral Polygons/Equiangular Polygons
These types of polygons have different features. If you want to know more about each polygon, join our maths tuition class at Miracle Learning Centre. In our maths tuition centre, we provide you with comprehensive knowledge.
Let's now examine the total number of angles in polygons.
The Sum of Interior Angles in a Polygon
One of the intriguing properties of polygons is that the sum of their interior angles follows a specific pattern. Regardless of the number of sides in a polygon, the sum of its interior angles remains constant. To find the sum of interior angles in a polygon, we can use the following formula:
Sum of Interior Angles = (n - 2) × 180°
In this formula:
- "n" represents the number of sides in the polygon.
- 180° is the measure of each interior angle in a triangle.
Now, let's apply this formula to different polygons to better understand how it works.
- Triangle (n = 3)
Sum of Interior Angles = (3 - 2) × 180° = 1 × 180° = 180°
- Quadrilateral (n = 4)
Sum of Interior Angles = (4 - 2) × 180° = 2 × 180° = 360°
- Pentagon (n = 5)
Sum of Interior Angles = (5 - 2) × 180° = 3 × 180° = 540°
- Hexagon (n = 6)
Sum of Interior Angles = (6 - 2) × 180° = 4 × 180° = 720°
- Octagon (n = 8)
Sum of Interior Angles = (8 - 2) × 180° = 6 × 180° = 1080°
As you can see, the sum of interior angles increases as the number of sides in the polygon increases. This consistent pattern holds true for all polygons, regardless of their shape or size.
The Sum of Exterior Angles in a Polygon
While we've discussed the sum of interior angles in polygons, it's equally important to explore the concept of exterior angles. Exterior angles in a polygon are formed by extending one side of the polygon outward. What's fascinating is that, unlike interior angles, the sum of exterior angles in any polygon always remains constant at 360 degrees, regardless of the number of sides.
To grasp this idea, let's consider a simple polygon, such as a triangle. Each exterior angle in a triangle is the supplementary angle to the corresponding interior angle. Since the sum of interior angles in a triangle is 180 degrees, each exterior angle measures 180 degrees minus the corresponding interior angle. This relationship holds true for all polygons.
For instance:
In a triangle, each exterior angle is 180° - 60° (interior angle) = 120°.
Consequently, the Sum of Exterior Angles = 3 × 120° = 360°.
This consistent pattern persists for all polygons, reinforcing the fundamental concept that the sum of exterior angles in any polygon is an unchanging value of 360 degrees.
Now, let's apply the concept of the sum of exterior angles to different polygons:
- Triangle (n = 3)
Each exterior angle is 180° - 60° (interior angle) = 120°.
Sum of Exterior Angles = 3 × 120° = 360°.
- Quadrilateral (n = 4)
Each exterior angle is 180° - 90° (interior angle) = 90°.
Sum of Exterior Angles = 4 × 90° = 360°.
- Pentagon (n = 5)
Each exterior angle is 180° - 108° (interior angle) = 72°.
Sum of Exterior Angles = 5 × 72° = 360°.
This consistent pattern holds true for all polygons, reinforcing the notion that the sum of exterior angles in any polygon is a fixed value of 360 degrees.
Real-life Application of Angles:
Understanding the sum of angles in polygons is not just a mathematical exercise. It has practical applications in various fields, such as architecture, engineering, and even art. Architects use polygonal angles to design buildings with precise angles and measurements. Engineers apply this knowledge in structural design to ensure stability. Even artists use geometric shapes and angles to create visually appealing compositions.
A good maths tuition can be of great assistance to students who want to understand angles in the context of everyday life. At our maths tuition Centre, Miracle Learning Centre, we are dedicated to assisting students in deciphering the geometry of angles and making connections between these concepts and everyday life.
Our knowledgeable teachers lead students through practical examples to make maths more interesting and applicable to their daily lives. Our maths tuition programme gives students the knowledge and abilities they need to succeed in both their academic endeavours and real-life obstacles, whether it be comprehending the angles in architectural drawings or understanding the principles of structure stability.
Conclusion:
The sum of angles in a polygon is a fascinating aspect of geometry that highlights the relationship between the number of sides and the total interior angle sum. Understanding this principle allows us to find the sum of interior angles in polygons of various shapes and sizes. Whether you're solving mathematical problems, designing structures, or simply exploring the beauty of geometry, this knowledge of polygons and their interior angles is a valuable tool.
If you aspire to explore the vast world of mathematics further, our Maths Tuition Centre is the perfect place to embark on your journey to mathematical excellence. With expert instruction, a comprehensive curriculum, and a commitment to your success, our tuition center will help you unlock the potential within you and open doors to a future filled with mathematical achievements. Take action now and join us to begin your journey to a better mathematical future!