Probability, often regarded as the backbone of statistics, is a fascinating branch of mathematics that enables us to understand and predict uncertainty in various scenarios. Whether it's predicting the outcome of a coin toss or assessing the likelihood of winning a game of chance, probability plays a crucial role in our everyday lives. In this blog, we embark on a journey to demystify the concepts of probability, exploring its significance, basic principles, and real-world applications. At Miracle Learning Centre, we believe maths tuition will empower students with enhanced skills in mathematics.
Understanding Probability
Probability, at its core, is the study of uncertainty and randomness. It provides a framework for quantifying the likelihood of different outcomes in a given event. The probability of an event is expressed as a value between 0 and 1, where 0 indicates impossibility, 1 indicates certainty, and values in between represent varying degrees of likelihood. We employ interactive and engaging teaching methods to help students grasp these fundamental concepts, making probability an accessible and intriguing subject.
How to Calculate Probability?
The probability of an event A is calculated using the following formula:
P(A)= (Number of favorable outcomes for A)/(Total number of possible outcomes)
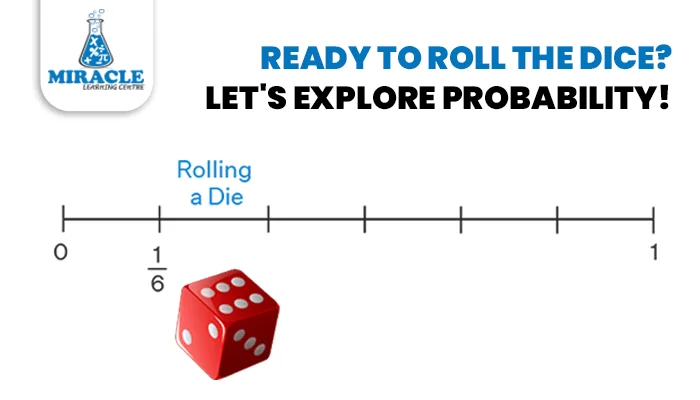
This formula reflects the basic idea that probability is a ratio of the number of outcomes favorable to the event of interest to the total number of possible outcomes. For example, if we are rolling a fair six-sided die and want to find the probability of rolling a 3, the formula would be:
P(Rolling a 3)=1/6
Examples
Let's delve into a couple of examples to better understand how probability works.
Example 1: Coin Toss
Consider a fair coin. The possible outcomes are either heads (H) or tails (T). The probability of getting heads can be calculated as:
P(Heads)= (Number of heads outcomes)/(Total possible outcomes)= 1/2
This implies that, in a fair coin toss, the chance of getting heads is 50%.
Example 2: Deck of Cards
Suppose you have a standard deck of 52 playing cards. What is the probability of drawing an Ace? The formula gives us:
P(Drawing an Ace)= (Number of Aces)/(Total number of Cards)= 4/52
Simplifying, we find that the probability of drawing an Ace is 1/13.
Different Types of Probability
Probability can be classified into different types based on the nature of the events involved:
a. Marginal Probability:
This type of probability focuses on the probability of a single event occurring independently. For instance, the probability of rolling a 4 on a fair six-sided die is a marginal probability.
b. Joint Probability:
Joint probability deals with the likelihood of two or more events happening simultaneously. If we want to find the probability of rolling a 3 and flipping a coin to get heads, we would use joint probability.
c. Conditional Probability:
Conditional probability is the probability of an event occurring given that another event has already occurred. Mathematically, it is expressed as P(A∣B), where A is the event of interest, and B is the condition.
d. Independent and Dependent Events:
Events are independent if the occurrence of one event does not affect the occurrence of another. On the contrary, events are dependent if the occurrence of one event affects the occurrence of another.
Conclusion
As we conclude our exploration of probability, it's evident that this branch of mathematics holds the key to understanding uncertainty and making informed decisions. Miracle Learning Centre takes pride in its commitment to providing quality maths tuition centre, ensuring that students not only grasp the intricacies of probability but also develop a lifelong love for mathematics. Through a combination of experienced educators, engaging teaching methods, and a focus on real-world applications, we equip students with the tools they need to excel in mathematics and beyond. Join us on this exciting journey of discovery, where the world of probability unfolds, and possibilities become probabilities. To know more about probability, check out our website.